Thursday, March 27, 2008
Work Class/Team Stuff Day
Here's a link to a slide show of some really cool math tricks that will make everyone think you're a genius! (As if they didn't already know that.)
http://www.slideshare.net/simonesu/amazing-math-trick
Good luck to Cramer's team in Team Stuff Day!
Have a great Spring Break everyone!
Bethany
Tuesday, March 25, 2008
Logarithm Laws
After this largely unsuccessful attempt we dived head long into logarithm laws which are largely based on rules for exponents that we already should know.
Exercise 22 1-14 was assigned and don't forget about the 19 accelerated math objectives due on thursday






 http://www.swop.net/
http://www.swop.net/
adding to Amy's post
He began with explaining to us what a log was, and it is really just another type of notation that can be used to express things such as really big numbers and it is the inverse of an exponential function.
He also explained what the parts of a logarithmic function were.
In the function:
x = b^y --> x is the argument, b is the base, and y is the argument.
In the second screen shot, he then went on to show us how to write exponential funtions in logarithmic notation and vice versa.
In the third screen shot he showed us a logarithmic function that does not exist. This is because if you try to sub in a +2 for the x value you wont arrive at a -4 and if you sub in a -2 for x, you will end up with a +1/2
On the fouth and fifth slides he graphed examples of logarithmic functions, and on the following slide he gave us a list of 8 common log properties.
Exercise 20 #'s 1-12 and Exercise 21 #'s 1-8 are due for tomorrow!
Hope these notes were helpful and have a great day!

Anna
Monday, March 24, 2008
LOGs
Well, since there will be more people than just me putting stuff up today, I'll keep it short and sweet. You can hear what they have to say. Later!
Thursday, March 20, 2008
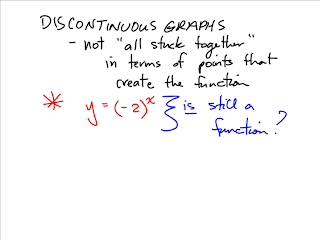
Exponential Functions
So today Mr. Max taught us a few things on Exponential Functions, beginning with identifying some of the parts of a function and some useful terms that we should iNvEsTiGaTe:

So here she is:
1. Find exact value of sin75.
sin(30+45) = (sin30*cos45)+(cos30*sin45)
= [(1/2)*((sqrt.2)/2))]+[((sqrt.3)/2)*((sqrt.2)/2)]
= ((sqrt.2)/4)+((sqrt.6)/4)
= ((sqrt.2)+(sqrt.6))/4
2. Simplify sin5xsin3x- cos5xcos3x.
= -(cos5xcos3x+sin5xsin3x)
= - cos(5x+3x)
= -cos8x
Better Late Than Never
Example One: Determine the exact value of sin135
sin(90+45)=sin90 cos45 + cos90 sin45
= (pi/2)(sqrt.2/2) + (0)(sqrt.2/2)
=(sqrt.2)(pi)/4
Example Two: Simplify: sin6xsin4x - cos6xcos4x
= -(cos6xcos4x-sin6xsin4x)
= -cos(6x+ 4x)
= -cos10x
Amy
Wednesday, March 19, 2008
Wednesday, March19
 rinkworks.com/graffiti/g/math.png
rinkworks.com/graffiti/g/math.pngThat is a true mental breakdown.(I couldn't get the picture to appear clearer)
 www.saynotocrack.com/img/geometry/question3.gif
www.saynotocrack.com/img/geometry/question3.gifThis one also made me laugh.
I hope you enjoyed my random post.
Tuesday, March 18, 2008
identities sum and differences
So hear is exhibit A using the identity Cos(a+b):
= Cos(A)* Cos(B) -Sin(A)*Sin(B)
= Cos(5x)*Cos(2x)-Sin(5x)*Sin(2x)
= Cos(5x+2x)
= Cos(7x)
And now for exhibit B which is like A in reverse and more specific:
Cos(75 degrees)
= Cos(45 degrees+ 30 degrees)
= Cos(45)*Cos(30)-Sin(45)*Sin(30)
= (sqr(2)/2) * (sqr(3)/2) - (Sqr(2)/2) * 1/2
= (sqr(6)/4) - (sqr(2)/4)
= (sqr(6)-sqr(2))/4
Well hope this helps someone
He also told us that the test will be the same as the pre-test, but with different numbers. Since the whole pre-test idea seems to be liked by the whole class, we will continue to get pre-tests before each of our real bi-weekly tests.
One last thing-The latest due date for Accelerated Math is March 27th with 19 objectives.
Well good luck on the test tomorrow everyone!
Amy

from my.opera.com/offspring/blog/show.dml/359734
Double Angle Identities
Sorry this post is a little late.. I never had time yesterday and today it took me awhile to upload images due to the sweet sun computers.
Anywho, today Mr. Maksymchuk continued on his journey to explain identities. Today we did some more work with double angle identities. As shown below, Mr. Max took all the equations given to us in our exam formula sheet and showed how they derived the formula. I found this part very helpful and makes learning identities easier to understand!
 Another things that I found useful in today's lesson is to always keep in mind the key triangles that we learned at the start of the year. Also take not of a 30, 60, 90 triangle with lengths of 3, 4, and a hypotenuse of 5. Knowing these triangles will come in very handy.
Another things that I found useful in today's lesson is to always keep in mind the key triangles that we learned at the start of the year. Also take not of a 30, 60, 90 triangle with lengths of 3, 4, and a hypotenuse of 5. Knowing these triangles will come in very handy. In this picture below, they wanted to know the value of 2(theta). So Max simply input the values for theta into another formula from our exam formula sheet, and solved for cosine and sine. Always note the signs you apply to the final answer so you make sure you have it in the right quadrant.
In this picture below, they wanted to know the value of 2(theta). So Max simply input the values for theta into another formula from our exam formula sheet, and solved for cosine and sine. Always note the signs you apply to the final answer so you make sure you have it in the right quadrant. Below is just another example of solving double angles using exact values. Remember to FACTOR out like terms!!!
Below is just another example of solving double angles using exact values. Remember to FACTOR out like terms!!!

Well this is the end of my post. Any questions or comments on how I can improve PLEASE let me know. Thank you for your time.
Remember!!! Always keep the stick on the ice.
Love Cramer
Friday, March 14, 2008
Pi Day!!!
Today's lecture was short as Mr. Maksymchuk decided that we should use the period to work on the pre-test and catch up with accelerated math. Employing the sum and difference identities that were taught to us yesterday, Maksymchuk showed us how to derive sleek-and-sexy numerical values for trigonmetric functions with"strange" angles, among other things:


Here he derived an equivalent expression for sec(θ-π/4):
Continuing with the sleek-and-sexy values:
And finally we were given our assignment:

Mr. Maksymchuk finished this around 12:30 p.m., giving us about 40 minutes to work on our pre-test and finish up our accelerated math.
Idenities Example
1) Find the exact value of the following:
a. sin (π/12) =sin (π/3-π/4) = sin(π/3) cos(π/4) - cos(π/3) sin(π/4)
= (√3/2)(√2/2) - (1/2)(√2/2)
= (√6/4) - (√2/4)
final answer = (√6 - √4)/4
2) Solve for "x" where 0° ≤ x ≤ 360°
a. sin2(x) = sin(x)
2sin(x) cos(x) = sin(x)
2sin(x) cos(x) - sin(x) = 0
sin(x) (2cos(x) - 1) = 0
Using identities (sum and difference examples)
βεθany was nice enough to help me out!!
Example 1:
Simplify: sin6x sin4x - cos6x cos4x
(for this you will have to use the identity) :
cos(α+β)= cosαcosβ - sinαsinβ
sin6x sin4x - cos6x cos4x =
-(cos6xcos4x - sin6xsin4x) = -cos(6x+4x) =
-cos10x
Example 2:
Determine the exact value of sin 75°
To begin, use the identity: sin(α+β) = sinαcosβ
sin(30+45) =sin 30cos 45 + cos 30 sin 45
= 1/2(√2/2) + √3/2(√2/2)
=√2/4 + √6/4
=√6+√2 /4
Trigonometric Sum and Difference Example Post
1). Find sin (60º-45º)
Employing sin(a-b) = sin(a)cos(b) - sin(b)cos(a)
sin(60º-45º) = sin(60º)cos(45º) - sin(45º)cos(60º)
=(√(3)/2)(√(2)/2) - (√(2)/2)(1/2)
=(√6 - √2)/4
2). Find cos(-x)
Employing cos(2π-x) = cos(-x) and cos(a-b) = cos(a)cos(b) - sin(a)sin(b) we have:
cos(-x) = cos(2π-x)
= cos(2π)cos(x) - sin(2π)sin(x)
= (1)cos(x) - (0)sin(x)
= cos(x)
Voilà!
Thursday, March 13, 2008
Sum and Difference Identity Examples
Example 1
Simplify: sin4xcos3x - cos4xsin3x
By using the identity sin(a - b) = sinacosb - cosbsina,
sin4xcos3x - cos4xsin3x = sin(4x - 3x)
= sin(x)
Example 2
Determine the exact value of cos(315). (That would be 315 degrees, but I don't know how to get a degrees sign on here either.)
By using the identity cos(a - b) = cosacosb + sinasinb,
cos(360 - 45) = cos360cos45 + sin360sin45
= 1(SQRT(2)/2) + 0(SQRT(2)/2)
= SQRT(2)/2
OR
By using the identity cos(a + b) = cosacosb - sinasinb,
cos(270 + 45) = cos270cos45 - sin270sin45
= 0(SQRT(2)/2) - (-1)(SQRT(2)/2)
= -(-1SQRT(2)/2)
= SQRT(2)/2
Hopefully that makes some sort of sense. Don't forget, tommorow's pi day!!
The following slides are from todays lesson.....
Wednesday, March 12, 2008
Wednesday, March 12

If the left hand side (LHS) equals the right hand side (RHS) then the identity is proved to be true. The correct terminology is shown in the slide below
 In order to master the art of proving identities you must practice practice practice! To some, (like myself) it can be very difficult and frustrating which is why it is important to keep up with your exercises and practice.
In order to master the art of proving identities you must practice practice practice! To some, (like myself) it can be very difficult and frustrating which is why it is important to keep up with your exercises and practice. It is important to know that to verify and to prove are similar but not the same. When you are verifying something you finding an identity for a specific case but it does not prove the identity for the whole equation.
It is important to know that to verify and to prove are similar but not the same. When you are verifying something you finding an identity for a specific case but it does not prove the identity for the whole equation.

Similarily to graphing, verifying cannot be used to prove an identity but you can use it to disprove.


Together we went through a few different problems for proving identities which are shown in the above slides.
Even though today was our deadline for accelerated math, Mr. Max was ever so kind to give us until friday to get done our first nine objectives. So don't forget to get those done by 3:40 friday and for tomorrow have questions 1-9 of exercise 15 to complete.
Thats all for now, so remember, keep your eye on the ball!
By the way happy birthday Cindy Lou!!! Hope you have a good one!

Picture courtesy of "http://www.freerangechick.com/images/bdayCard/BBigBirthdayCake.jpg"